SHM-Simple Harmonic Motion

Simple Harmonic Motion

A particle is said to move with S.H.M when the acceleration of the particle about a fixed point is proportional to its displacement but opposite in direction.

Hence, when the displacement is positive the acceleration is negative(and vice versa).
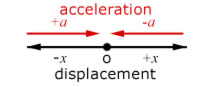
This can be described by the equation:

where x is the displacement about a fixed point O(positive to the right, negative to the left), and w² is a positive constant.
An equation for velocity is obtained using the expression for acceleration in terms of velocity and rate of change of velocity with respect to displacement(see 'non-uniform acceleration').

separating the variable and integrating,

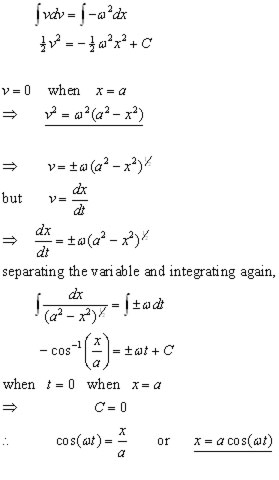
NB cos^-1() is the same as arc cos()

So the displacement against time is a cosine curve. This means that at the end of one completete cycle,

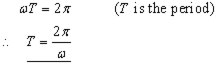
Example

A particle displaying SHM moves in a straight line between extreme positions A & B and passes through a mid-position O.

If the distance AB=10 m and the max. speed of the particle is 15 m^-1 find the period of the motion to 1 decimal place.